Bubbletons in hyperbolic 3-space and spherical 3-space

Author

Description

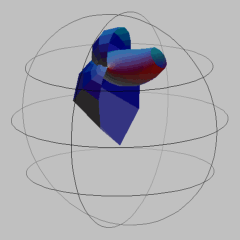
We show the cylinder bubbletons in hyperbolic 3-space and spherical 3-space, which are constant mean curvature H 1 and constant mean curvature H 0 surfaces, respectively. We constructed these surfaces using the DPW method (see [1]).

Bubbletons in Euclidean 3-space have been closely examined in [3]. In this model, we show bubbleton surfaces in hyperbolic 3-space and spherical 3-space using the DPW method. In the Euclidean case, bubbleton surfaces are CMC surfaces made from Backlund-Bianchi transformations of the round cylinder. Such a surface is shaped like a cylinder with attached bubbles, thus it is called a bubbleton.

The parallel constant positive Gaussian curvature surface of a bubbleton in Euclidean 3-space is well known and was first found by Sievert, thus it is called the Sievert surface.

With respect to the DPW method (see [1]), the Backlund transformation is a dressing action on loop groups and this dressing action is described by elements of the simplest possible type like those of Terng and Uhlenbeck [4].

The DPW method has natural extensions for constructing constant mean curvature H 1 and H 0 surfaces in hyperbolic 3-space and spherical 3-space, respectively. Thus we can do the "Backlund-Bianchi transformation" (as a dressing action similar to the Euclidean 3-space case) for constant mean curvature H 1 and H 0 surfaces in hyperbolic 3-space and spherical 3-space, respectively.

We can give explicit parametrizations of the cylinder bubbletons in hyperbolic 3-space and spherical 3-space. In this model, the bubbleton in hyperbolic 3-space is shown using the Poincare ball model for hyperbolic 3-space, and the bubbleton in spherical 3-space is shown using stereographic projection of spherical 3-space. (Only half of the surfaces are shown here, and the halves not shown can be obtained by a single reflection of the halves that are shown.) These bubbletons in hyperbolic 3-space and spherical 3-space have also been constructed by Nick Schmitt using the CMC Lab software (see [2]). Detailed explanations of these explicit parametrizations can be found in the postscript, pdf and Mathematica files included in this model. The specific parameters used to compute the Master model files are described in the Mathematica files. Basic introductory material is also included in the postscript and pdf files.

Model produced with: JavaView v.2.13.002

References

1. J. Dorfmeister, F. Pedit and H. Wu: Weierstrass type representation of harmonic maps into symmetric spaces, Comm. Anal. Geom. 6, 4 (1998), 633--668, .
2. N. Schmitt: CMCLab software, http://www.gang.umass.edu/gallery/cmc.
3. I. Sterling and H. C. Wente: Existence and classification of constant mean curvature multibubbletons of finite and infinite type , Indiana U. Math. J. 42, 4 (1993), 1239--1266, .
4. C.-L. Terng and K. Uhlenbeck: Backlund transformations and loop group actions, Comm. Pure and Appl. Math. 53 (2000), 1-75, .

Files

• Master File: H3bubbleton.jvx
• Master File: S3bubbleton.jvx
• Applet File: H3bubbleton.jvx
• Applet File: S3bubbleton.jvx
• Preview: H3bubbleton.gif
• Preview: S3bubbleton.gif
• Other: H3bubbleton.nb
• Other: S3bubbleton.nb
• Other: bubbleton.pdf
• Other: bubbleton.ps

Submission information

Submitted: Mon Mar 4 04:34:15 GMT 2002.
Revised: Tue Oct 1 14:53:31 CEST 2002, Mon Jan 6 17:48:13 GMT 2003.
Accepted: Fri Feb 14 18:06:11 CET 2003.

Author's Address

Kobe University
Mathematics Department
Faculty of Science
Rokko, Kobe 657-8501
Japan
kobayasi@math.kobe-u.ac.jp
http://www.math.kobe-u.ac.jp/~kobayasi