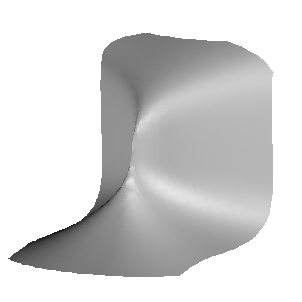
Zero set of x^3 - y^5 - z^2, the E8 singularity

Author

Description

Zero set of function f(x,y,z) = x^3 - y^5 - z^2: the E8 singularity for function from R^3 to R.

This model is one of a collection of the zero sets of singularities of functions from R³ to R.

This model shows the zero set f^-1(0) for the E₈ singularity: f(x,y,z) =x³-y⁵-z².

Singularities of functions from Rⁿ to R were clasified by V.I. Arnold [1], an introduction to singularity theory can be found in [2]. The models were calculated using the Liverpool Surface Modelling Package [3,4,5] and JavaView [6].

Model produced with: Liverpool Surface Modeling Package 2.9 and JavaView

References

1. V. I. Arnold: Singularity Theory, London Mathematical Society (1981).
2. J. W. Bruce and P. J. Giblin: Curves and Singularities, Cambridge University Press (1992).
3. R. J. Morris: A new method for drawing Algebraic Surfaces, in R. B. Fisher (Eds.): Design and application of curves and surface, Oxford University Press (1994), 31-48.
4. R. J. Morris: The Use of Computer Graphics for Solving Problems in Singularity Theory, in H-C Hege, K Polthier (Eds.): Visualization and Mathematics, Springer (1997), 53-66.
5. R. J. Morris: Algebraic Surfaces Web-Server, http://www.comp.leeds.ac.uk/pfaf/lsmp/AsurfClient.html.
6. K. Polthier, S. Khadem-Al-Charieh, E. Preuss and U Reitebuch: JavaView, http://www.javaview.de/.

Files

• Master File: E8mm_Master.jvx
• Applet File: E8mm_Applet.jvx
• Preview: E8mm_Preview.gif

These files have been hand edited to ensure that the boundaries are correct and that they are topologically correct around the singular point.

Submission information

Submitted: Mon Jun 25 13:17:04 MDT 2001.
Revised: Fri Apr 19 18:21:54 MDT 2002.
Accepted: Mon Sep 16 13:32:22 CEST 2002.

Author's Address

Department of Statistics, University of Leeds
Department of Statistics,
University of Leeds,
Leeds, LS2 9JT
England
rjm@amsta.leeds.ac.uk, webmaster@pfaf.org
http://www.amsta.leeds.ac.uk/~rjm/