Octahedron EG-Models Home

image octahedron_Preview.gif
Electronic Geometry Model No. 2001.02.056

Author

Martin Henk

Description

Densest lattice packing of an octahedron

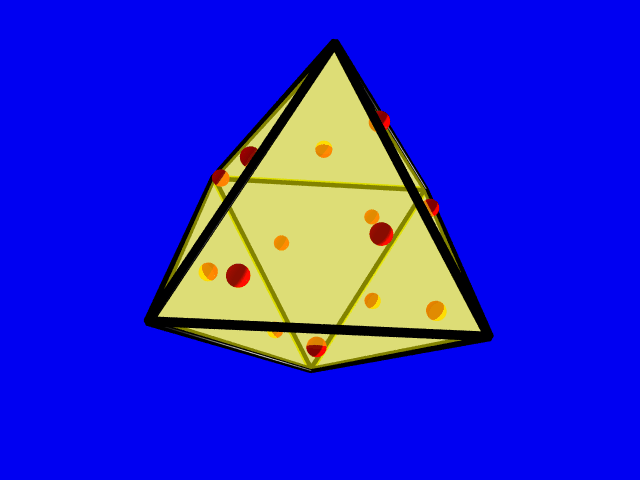
The octahedron has 6 vertices, 12 edges and 8 triangular facets. It is one of the five Platonic solids (it represents the element air in Plato's Timaios) and its dual is the cube.

The density of a densest lattice packing of an octahedron was already calculated by Minkowski in 1904. In 1948 Whitworth generalized Minkowski's result to a family of truncated cubes. The density of a densest lattice packing is equal to 18/19 = 0.9473..., and the 14 points in the picture show the lattice points of a critical lattice lying in the boundary of an octahedron.

Model produced with: JavaView v2.00.a11

Keywords lattice packings; polytopes; packings; critical lattice; octahedron
MSC-2000 Classification 52C17 (11H31)
Zentralblatt No. 01682997

References

  1. Ulrich Betke and Martin Henk: Densest lattice packings of 3-polytopes, Comp. Geom. 16 , 3 (2000), 157 - 186.
  2. Hermann Minkowski: Dichteste gitterförmige Lagerung kongruenter Körper, Nachr. K. Ges. Wiss. Göttingen, Math.-Phys. KL (1904) (1904), 311 - 355 (see also Gesammelte Abhandlungen vol. II, 3 - 42, Leipzig 1911).
  3. J.V. Whitworth: On the densest packing of sections of a cube, Annali Mat. Pura Appl. 27 (1948), 29 - 37.

Files

Gif-file was produced by Povray 3.02

Submission information

Submitted: Thu Feb 1 16:41:52 CET 2001.
Accepted: Fri Apr 27 14:11:54 CET 2001.

Author's Address

Martin Henk
University of Magdeburg
Department of Mathematics
Universitätsplatz 2
D-39106 Magdeburg
henk@mail.math.uni-magdeburg.de
http://www.math.uni-magdeburg.de/~henk